Math
| Responsible | Andy Hakim |
|---|---|
| Last Update | 05/07/2025 |
| Completion Time | 2 days 3 hours 16 minutes |
| Members | 1 |

How Giving Up Reality Solved the Cubic Equation
For centuries, a general solution to the cubic equation was considered impossible—until mathematicians embraced complex numbers, abandoning the strict requirement that math reflect physical reality.
References:
- Videos:
-
Books and Papers:
- Dunham, W. (1990). Journey Through Genius: The Great Theorems of Mathematics. Link
- Toscano, F. (2020). The Secret Formula, Princeton University Press. Link
- Bochner, S. (1963). The Significance of Some Basic Mathematical Conceptions for Physics, Isis, 54(2), 179–205. Link
- Muroi, K. (2019). Cubic Equations of Babylonian Mathematics, arXiv:1905.08034. Link
- Branson, W. (2014). Solving the Cubic with Cardano. Link
- Rothman, T. (2013). Cardano v Tartaglia: The Great Feud Goes Supernatural, arXiv:1308.2181. Link
- Siadat, M. V., & Tholen, A. (2021). Omar Khayyam: Geometric Algebra and Cubic Equations, Math Horizons, 28(1), 12–15. Link
- Merino, O. (2006). A Short History of Complex Numbers, University of Rhode Island. Link
-
Historical Works:
- Cardano, G. (1545). Ars Magna (Dover reprint, 1993). ISBN: 0-486-67811-3
- Bombelli, R. (1579). L’Algebra. Link
-
Software:
- Manim Community Developers. (2021). Manim – Mathematical Animation Framework (v0.13.1). Link
Academic
View allStudent Content
View allExtra
View all
How Giving Up Reality Solved the Cubic Equation
For centuries, a general solution to the cubic equation was considered impossible—until mathematicians embraced complex numbers, abandoning the strict requirement that math reflect physical reality.
References:
- Videos:
-
Books and Papers:
- Dunham, W. (1990). Journey Through Genius: The Great Theorems of Mathematics. Link
- Toscano, F. (2020). The Secret Formula, Princeton University Press. Link
- Bochner, S. (1963). The Significance of Some Basic Mathematical Conceptions for Physics, Isis, 54(2), 179–205. Link
- Muroi, K. (2019). Cubic Equations of Babylonian Mathematics, arXiv:1905.08034. Link
- Branson, W. (2014). Solving the Cubic with Cardano. Link
- Rothman, T. (2013). Cardano v Tartaglia: The Great Feud Goes Supernatural, arXiv:1308.2181. Link
- Siadat, M. V., & Tholen, A. (2021). Omar Khayyam: Geometric Algebra and Cubic Equations, Math Horizons, 28(1), 12–15. Link
- Merino, O. (2006). A Short History of Complex Numbers, University of Rhode Island. Link
-
Historical Works:
- Cardano, G. (1545). Ars Magna (Dover reprint, 1993). ISBN: 0-486-67811-3
- Bombelli, R. (1579). L’Algebra. Link
-
Software:
- Manim Community Developers. (2021). Manim – Mathematical Animation Framework (v0.13.1). Link
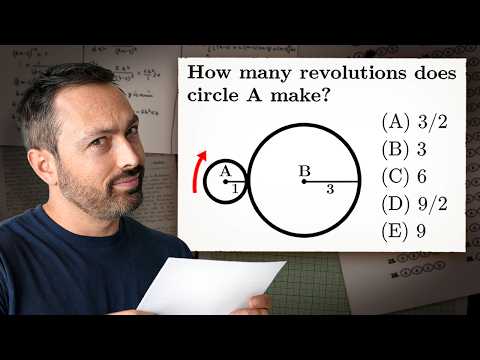
How an SAT Question Became a Mathematical Paradox
A fascinating breakdown of an SAT question that led to widespread confusion and uncovered a deeper paradox in mathematics.
References:
- MindYourDecisions. Summary of this problem: YouTube
- Kyle Hill. More cool math about this problem: YouTube
- MinutePhysics. Discussion of a solar day: YouTube
- Murtagh, J. (2023). The SAT Problem That Everybody Got Wrong, Scientific American: Link
- United Press International (1982). Error Found in S.A.T. Question, New York Times: Link
- Yang (2020). Hardest SAT math problem?, Quora: Link
- Coin rotation paradox, Wikipedia: Link
- Simmons, B. (2015). Circle revolutions rolling around another circle, Math StackExchange: Link
- Sidereal time, Wikipedia: Link
- Solar Time vs. Sidereal Time, Las Cumbres Observatory: Link
- Zotti, G., et al. (2021). The Simulated Sky: Stellarium for Cultural Astronomy Research: Link
- Newspapers from 1980s–1990s, Newspapers.com: Link
- SAT Practice Test, College Board: Link
- Revolution Definition, NASA: Link
- Revolution Definition, Merriam-Webster: Link
- Earth motion animation, NASA: Link
- Satellite animation, NASA: Link

Einstein was wrong about black holes — what else?
This video was inspired by conversations with leading physicists and key scientific literature. Below are the references:
References
- Thorne, K. (1995) – Black Holes & Time Warps: Einstein's Outrageous Legacy
- Hamilton, A. J. S. (2021) – General Relativity, Black Holes, and Cosmology – Link
- Relativity Playlist by ScienceClic – Link
- Black Hole Events by PBS Space Time – Watch
- Newton’s Letters via The Newton Project – Link
- Einstein, A. (1915) – Die Feldgleichungen der Gravitation – Link
- Schwarzschild, K. (1916) – Über das Gravitationsfeld eines Massenpunktes – Link
- Wali, K. C. (1982) – Chandrasekhar vs. Eddington—An Unanticipated Confrontation – Link
- How to Build a Black Hole – Watch
- Oppenheimer, J. R., & Volkoff, G. M. (1939) – On Massive Neutron Cores – Link
- Oppenheimer, J. R., & Snyder, H. (1939) – On Continued Gravitational Contraction – Link
- Schwarzschild Geometry by Andrew Hamilton – Link
- Why Time and Space Swap by ScienceClic – Watch
- Why All World Maps Are Wrong by Vox – Watch
- Hamilton, A. J., & Lisle, J. P. (2008) – The River Model of Black Holes – Link
- Mapping The Multiverse by PBS Space Time – Watch
- Rotating Black Hole via Wikipedia – Link
- Wormhole Travel by PBS Space Time – Watch
- Morris, M. S., & Thorne, K. S. (1988) – Wormholes in Spacetime and Their Use for Interstellar Travel – Link
🖼 Visuals & Simulation References
- ScienceClic – “What would we see if we fell into a black hole” – Watch
- Kazmierczak, J. et al. (2021) – NASA’s NICER Tests Matter’s Limits – Link
- Bridgman, T. et al. (2024) – M5.1 Flare at Active Regions 13559 & 13561 – Link
- Schnittman, J. et al. (2019) – Black Hole Accretion Disk Visualization – Link
- Wiessinger, S. et al. (2020) – A Decade of Sun – Link
- Skelly, C. et al. (2017) – What is a Neutron Star? – Link
- Earth texture – Link
- First image of Sgr A* – Link
- Image of M87 – Link
- Polarized light image of Sgr A* – Link